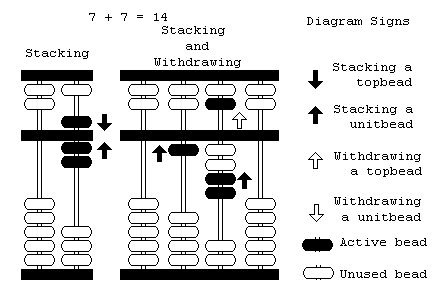

This is the diagram which ended the last section. We show how to add 7 to 7 and give the answer 14 above.
Firstly, seven is stacked by moving a topbead and two unitbeads to the crossbar.
Next we have to think differently about the seven we are about to add to the original number seven.
Seven can be thought of as 10 minus 3. ie, 7 = 10 - 3
Also, 3 can be thought of as 5 minus 2. i.e. 3 = 5 - 2
By going through two indirect routes, we arrive at the answer.
ie.
7 = 10 - (5 -2) = 10 - 5 + 2
That is, put a bead in the tens column, remove a topbead (5) and then add two unit beads.
Alternatively, the net result of this is that, 7 = 12 - 5, so we stack in 12, and remove five.
Due to the constraints of the abacus' design, doing this route is by far the most natural way to the answer. It is a basic Chinese abacus manipulation technique, which we call SUBSTITUTION. It is modified later when we see how it is used in subtraction.
Obviously if the two numbers add up to less than 10, so it fits on a rod, substitution is clearly not used.
will have 8 on the unit rod first, then the digit 1 (from the number 12) is placed into the tens column. The digit 2 is added to the units column, but we note it gives 10, so we carry a one over to the tens column and clear the units column. (ie., 2 = 10 - 8, so add a second bead to the tens rod, and then take away 8 from the units column)
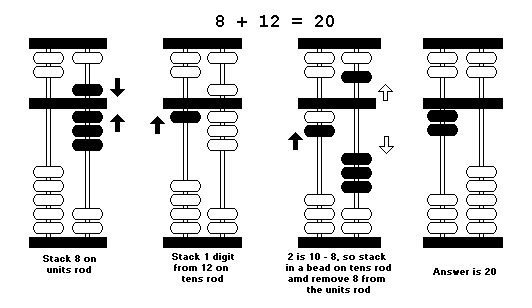
The next page will deal with the addition of numbers with many more digits.
© Dylan W.H.S. 1996-onwards
You can e-mail me here on anything you like!